Sum Star by Dan Adams
Theme: Logical
Author/Opus: This is the 1st puzzle from guest contributor Dan Adams.
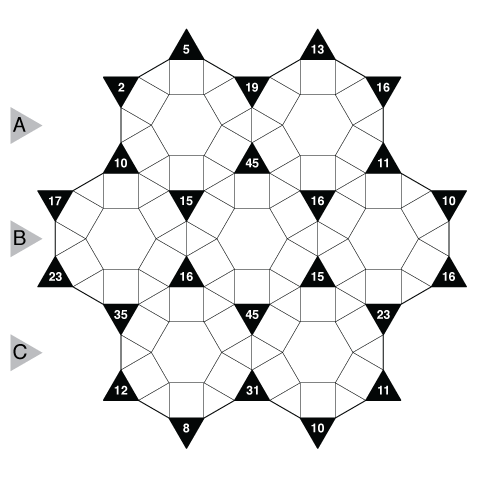
Rules: Place digits into some cells and shade all remaining cells so that: each dodecagon contains the digits 1-9 exactly once; digits in cells sharing a vertex with a black triangle add up to the indicated clue number without repeats; and shaded cells cannot share an edge with another shaded cell.
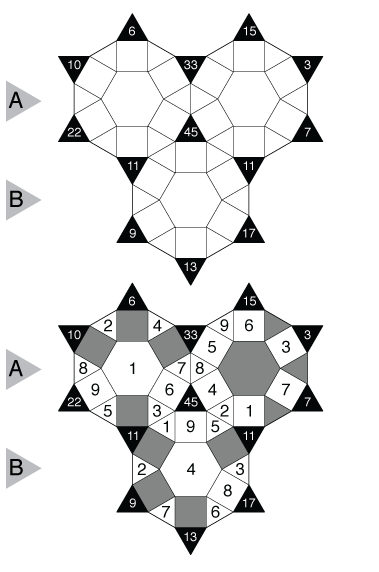
Answer String: Enter the digits in the marked central rows (triangle, hexagon, triangle, …). Use a capital X for shaded cells. Separate each row with a comma. The example has the key “8178XX,243”.
Time Standards (highlight to view): Grandmaster = 16:00, Master = 32:00, Expert = 1:04:00
Solution: PDF
Note: Follow this link for other less common Number Placement puzzles.

This was very hard, and that’s an understatement.
When we say: “digits in cells sharing a vertex with a black triangle add up to the indicated clue number without repeats”
do we mean that 1) a 7 may not touch any triangle twice 2) if a 7 touches a triangle twice, only include it in the sum once 3) something else?
Statement 1 is correct. No two cells sharing a vertex with a black triangle can have the same value.