Dr. Sudoku Prescribes #122 – Battleships (Square Ends)
or solve online (using our beta test of Penpa-Edit tools; use tab to alternate between ship placement and shading modes. In ship placement mode, right click gives sea, left click gives circle/square, left click and drag for rounded ships.)
This is a “Franken-Friday” puzzle variation.
Theme: Squares of Squares
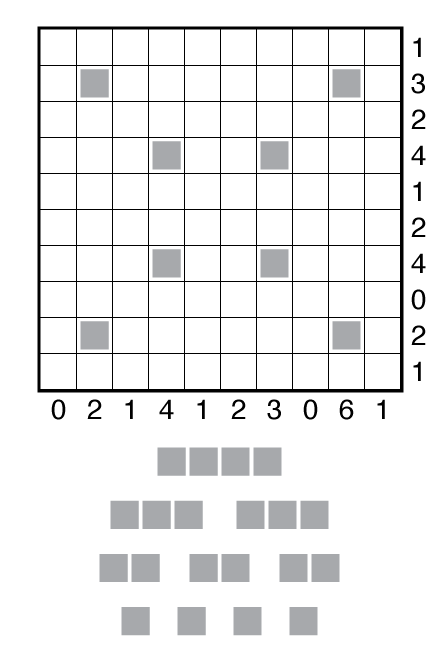
Rules: Standard Battleships rules. The fleet in this puzzle has no rounded ends; instead, all ship segments are square and indistinguishable.
Answer String: For each row from top to bottom, enter the number of the first column from the left where a ship segment appears. If the row is empty, enter 0. Enter these numbers as a single string with no separators.
Time Standards (highlight to view): Grandmaster = 3:30, Master = 5:45, Expert = 11:30
Solution: PDF

19 : 15 S .Placed the 4 – unit wrongly in the first 2 tries and then placed it correctly .
09:51.
Definitely a tough one, with a start of locating all three largest ships as usual. Took me a long time to remove many 4-ship possibilities; is that the intended path?
Anyway, neat puzzle. The gimmick is neat, with a similarly neat solving path.
There are four rows/columns that can hold the three largest ships, but both R4 and C4 require that ship to use the square clue in R4C4. So R7 must have such a big ship, which must include R7C5, and a narrow but logical path starts from there.
Yup, that’s exactly the way I did it (and it took me a loooong time to get there, because I really didn’t want to fumble my way through by guessing).
I wanted to see how the Fathom It! solver would fare with the original puzzles. The following is its method of solving the board.
To simplify things for us humans, we can first fill in the obvious waters:
1
1 2 3 4 5 6 7 8 9 0
+---------------------
A | _ _ _ . . . . _ . _ 1
B | _ * . . . . . _ * . 3
C | _ _ _ . _ _ . _ . _ 2
D | _ _ . * . . * _ . . 4
E | _ _ _ . _ _ . _ . . 1
F | _ _ _ . _ _ . _ . . 2
G | _ _ . * . . * _ . . 4
H | _ _ _ _ _ _ _ _ _ _ 0
I | _ O _ _ _ _ _ _ * _ 2
J | _ _ _ . . . . _ . _ 1
0 2 1 4 1 2 3 0 6 1
Row C has a count of 2 but has only three empty cells, so it’s a good place to start placing waters one at a time, looking for obvious contradictions.
(*) If we try to place a water in C4, we reach a contradiction:
(*) C7 and C10 are ship segments
(*) The two 3-segment cruisers must be placed in A9-C9 and D3-D5.
(*) The battleship cannot be placed anywhere
(*) Therefore C4 is a ship segment
The following steps are all forced:
(*) G3 is a segment.
(*) The battleship can be placed in only two places, D9 is the overlap square, so it is a segment.
(*) D6 is a segment
(*) C9 is a segment
(*) The battleship must go in A9-D9
(*) E4 is a segment
(*) The remaining cruiser must be in G3-G5
(*) The rest is obvious
Not as elegant as Tom’s initial logic, but a generic and straightforward way of solving it.